RESONANT KINETICS AND DRIVEN PHASE SPACE HOLES

Frequently, waves in plasmas are described by the fluid approximation. Such fluid modes have been studied extensively both experimentally and theoretically. On the other hand, nearly 50 years ago, Bernstein, Greene and Kruskal (BGK) predicted existence of a large class of purely kinetic, dissipationless waves in plasmas, but experimental realization of these BGK modes is known to be difficult. A significant progress in the field was reported in recent experiments and theory on excitation of large amplitude chirped-bucket BGK (CBGK) modes in pure electron plasmas. The CBGK modes were excited by capturing a group of plasma electrons in the tail of the velocity distribution into resonance with external potential oscillations and dragging the trapped particles into the bulk of the velocity distribution by slowly varying the frequency of the external perturbation. The relocation of a low density region in phase-space created a growing depression (hole) in the phase-space distribution of plasma particles as this region entered the bulk of the distribution. The CBGK modes are intrinsically nonlinear plasma density/field structures associated with these phase-space holes.

Finally, we have studied a new phenomenon of controlled creation and manipulation of a phase-space hole in a plasma, with its associated BGK mode, via adiabatic Cherenkov-type resonance.

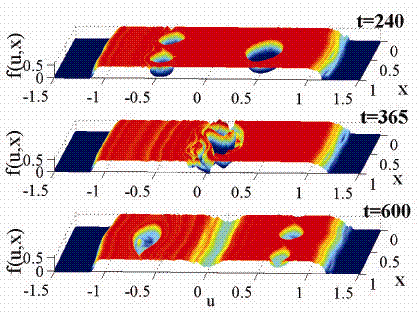
This figure shows an example of collision between two such BGK structures. The single and two-hole structures in this example were formed at opposite phase fluid boundaries by two resonant, varying frequency driving waves. Each structure is phase-locked to its drive forcing it to drift in velocity space until collision. After the collision, one can see reemergence of the two phase-locked structures in phase space.